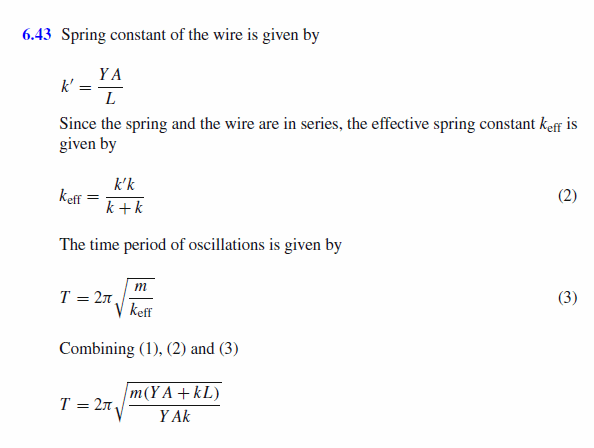
One end of a long metallic wire of length L is tied to the ceiling. The other end is tied to a massless spring of spring constant k. A mass m hangs freely from the free end of the spring. The area of cross-section and the Young's modulus of the wire are A and Y respectively. The mass is displaced down and released. Show that it will oscillate with time period T = 2pi·sqrt(m(YA+kL)/YAk) |
| New search. (Also 5349 free access solutions) |