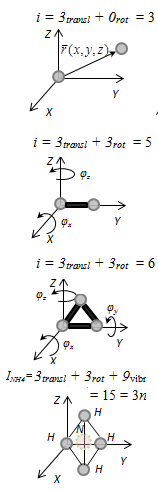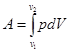PHYSICAL BASIS OF THERMODYNAMICS
1. The first law of thermodynamics

§ 1. The internal energy

Every thermodynamic system in any state has the energy, which is called the total energy. The total energy of the system consists of the kinetic energy of the system as a whole, the potential energy of the system as a whole and of the internal energy
  `
The internal energy of a system is the sum of all kinds of random (thermal) motion of molecules: the potential energy of the intratomic and intranuclear movements. The internal energy is a function of the gas state. For the internal energy of the gas state is uniquely determined, that is, a certain function.

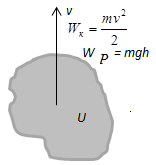
In the transition from one state to another internal energy changes. But the internal energy of the new state does not envy the process by which the system passed in this state.

§ 2. Heat and work

There are two different ways to change the internal energy of a thermodynamic system. The internal energy of the system can change as a result of the work and as a result of heat transfer system. The work is a measure of change in the mechanical energy of the system. When the work is a movement system or individual macroscopic parts relative to each other. For example, fitting-in piston in the cylinder, which houses the gas, we compress the gas, causing its temperature increases, ie changes the internal energy of the gas.
The internal energy can be varied as a result of heat transfer, ie impart to gas certain amount of heat Q.

The difference between the heat and the work is that the heat is transferred from a large number of microscopic processes in which the kinetic energy of the molecules of a heated body in collisions of molecules transferred less heated body. Common between heat and work, that they are functions of the process, that is, we can talk about the value of warmth and work, when the transition of the system from the state the first in the state of the second. The warmth and the work is not a function of the state, as opposed to the internal energy. We can not say what is the work and heat the gas in state 1, but the internal energy in the state 1 can talk.

§3 First law of thermodynamics

Suppose that a system (gas enclosed in a cylinder under the piston), having internal energy, get some heat Q, going into a new state, characterized by the internal energy of U₂, has made the work of A over the environment, that is, against the external forces. The amount of heat is positive when it is applied to the system, and negative when taken from the system. Work is positive when it is done with gas against external forces, and negative when it is done on the gas.

I Law of Thermodynamics: The amount of heat (ΔQ), the system is given to increase the internal energy of the system and to perform system work (A) against external forces.

Record the I thermodynamics beginning differentiall form

dU  - infinitesimal change of an internal energy of system

 - elementary work

 - infinitesimal amount of heat

If the system periodically returns to its original state, the change in the internal energy is equal to zero. Then

that is, I kind of perpetual motion machine, batch engine, which would have made a great job of it than the message from outside the energy is not possible (one their formulations I law of thermodynamics).

§2 The degrees of freedom of the molecule. The Law on the uniform
energy distribution of the molecule
Energy distribution on molecule degrees of freedom

       The number of degrees of freedom of the mechanical system is the number of independent variables, by which e can be specified at the system. Monatomic gas has three translational degrees of freedom i = 3, because to describe the position of the gas in the space of only three coordinates (x, y, z).

Rigid connection called a relationship in which the distance between the atoms is not changed. Diatomic molecule with a rigid connection (N₂, O₂, Н₂) have three translational degrees of freedom and two rotational degrees of freedom: i=i_transl +i_rot=3 + 2=5.

Translational degrees of freedom associated with the motion of the molecule as a whole in space, rotational - with rotation of the molecule as a whole. Rotation about the axes x and z by the angle  φ_x and φ_z  to changes in the position of the molecules in space, the rotation axis of the molecule does not change its position, therefore, coordinate φy in this case is not necessary. Triatomic molecule with a rigid connection has 6 degrees of freedom

i=i_пост +i_вр=3 + 3=6

If the bond between the atoms is not tough, it adds the vibrational degrees of freedom. For non-linear molecules і_vibr = 3N - 6, where N - number of atoms in the molecule.

Regardless of the total number of degrees of freedom of the molecules of 3 degrees of freedom is always progressive. None of the translational has no advantage over the other, so each of them is on average the same energy, equal to 1/3 of

       Boltzmann law has established that in order for the statistical system (ie, for a system in which large number of molecules), which is in thermal equilibrium at each translational and rotational degrees of freedom have an average kinematic energy equal to 1/2 kT, and for each vibrational degree of freedom - the average energy equal to kT. Vibrational degree of freedom "has" twice as much energy because it accounts for not only the kinetic energy (as in the case of the translational and rotational motion), but the potential energy, and  . so the average energy of a molecule

    We will consider a molecule with a rigid connection, so

as in an ideal gas the mutual potential energy of the molecules is zero (the molecules do not interact with each other), the internal energy is the product of 1 mol of the mean energy of one molecule to the number of molecules in a mole of substance, that is the number of Avogadro

§3 Heat capacity. Work gas

   1. Specific heat capacity of a substance - the value of which is equal to the amount of heat required to heat 1 kg of matter at 1K.

    Molar heat capacity - the value of which is equal to the amount of heat needed to heat one mole of a substance by 1K.

    Relationship molar and the specific heat

     Distinguish the specific heat at constant volume C_V (V = const) and constant pressure C_p (p = constif in the process of heating a substance to the volume or the pressure is kept constant.

• Work of gases at change of its volume

      Consider a gas in a piston and a cylinder

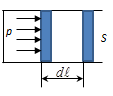
If the gas expands, the piston moves to the infinitesimal distance dl, the gas produced on the piston work.

Where S - the piston area. – change the volume of the system

 A total work done by the gas at the change in volume fromV₁ to V₂ is equal

• work for any process

3 C_p, C_V and the relationship between them (Mayer's equations) 

       Let's write down expressions I of the I law of thermodynamics for 1 mole gas

If the gas is heated at constant volume (V = const, dV = 0) A = 0, and imparted to the gas heat goes only to increase its internal energy

that is, the molar heat of the gas at constant volume equals the change in internal energy of one mole of gas at temperatures hanging 1K.

Because

If the gas is heated at constant pressure p = const

because  it does not depend on the type of process (the internal energy does not depend on p and V, is determined only by the temperature T) and

       From equation Mendeleev-Clapeyron

 - Mayer equation

       Mayer equation shows that C_p is always greater than the value of C_v on universal gas constant R, since at p = const requires an additional amount of heat to perform the work of expansion of gas, as constant pressure to ensure increased gas

Value