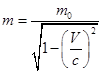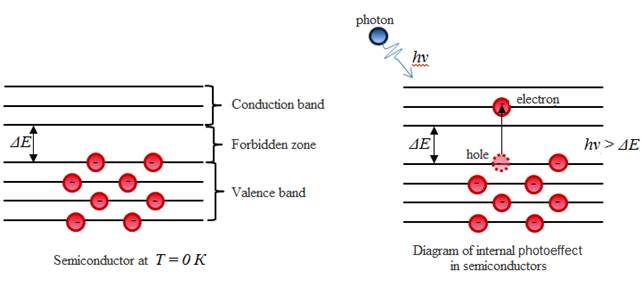
§4 Internal photoelectric effect. Photocells

Internal photoelectric effect - the redistribution of the electron energy levels in insulators and semiconductors (but not metal) under the action of light. If the photon energy hv of the incident light exceeds the band gap in an insulator or semiconductor, the electron absorbs a photon, is transferred from the valence band to the conduction band. As a result of this transition, formed the couple choices: the electron in the conduction band and the valence band - hole. Thus, in the conduction carriers appear, and when the semiconductor circuit on her current flows, or application of an external electric field current flows, which changes depending on the light.

Internal photoelectric effect leads to:

1) the carrier density in the conduction band (ie, change in conductivity);
2)  The emergence of the photo emf.

On the use of the internal photoeffect action is based solar cells - devices that convert light energy into electrical energy, or change their properties under the action of the incident light.
Changes the properties of photoconduction work on: photoresistor (PR), photodiodes (PD), phototransistors (PT), photoresists, photo chip. Optoelectronic pair - in one case concluded the light source and light detector - used for electrical isolation circuits.

A device that converts light energy into electrical energy using barrier-layer photoeffect (a kind of internal photoelectric effect) - the emergence of the photo emf on the pn junction or on the border of metal and a semiconductor. Deviceon barrier-layer photoeffect used in the cameras, in solar cells, calculators, on satellites, in some homes. Photocells are also used in photometry, spectroscopy, astrophysics, biology, etc.

Photoemission is used in vacuum photocells, photomultipliers, the vidicon (tube TV - and video cameras), etc.

Mass and the momentum of the photon. Light pressure

1) Photon - is a quantum of light. According to Einstein's hypothesis of light quanta, emission, absorption and distribution of light occurs in discrete portions (quanta) called photons (photo - light). The energy of a photon:

Einstein derived a formula that relates the mass and energy. Einstein formula:

For a photon Е = Е₀, therefore . Hence the mass of the photon:

Photon different from macroscopic bodies and elementary particles in that it is an elementary particle of light, which in any environment is moving at the speed of light and has no rest mass m_0photon = 0. The rest mass - a mass, which has a particle at V = 0, thus, there is no resting photons. If the light is to stop, then it means that the light energy is absorbed by the material and the light not will be. Photon mass should be considered as a field mass, which means that light has mass is related to the elementary field of the light wave. Photon has energy, but all energy corresponds weight (this follows). If understood by E the energy of the electromagnetic field, it should be understood by the mass m of the electromagnetic field of the light wave, thus, the field, as the substance has the energy and mass. Field - a form of existence of matter. The presence of the field of energy and mass is proof of the materiality of the electromagnetic field.

2) In addition to the energy and mass, the photon has a momentum P. In the general theory of relativity a relation between energy and momentum:

where с = 3 · 10⁸ m/s,

m₀ - rest mass, as for photon m₀ = 0, then. Е =ср, therefore,

From the above it follows that the photon, as well as any other particle has the energy, momentum and mass. These corpuscular characteristics of the photon associated with wavelengths of light - frequency:

The manifestation of the wave-particle duality of light - light is a wave and a particle.

Experimental proof of the existence of the photon momentum is light pressure. Radiation incident on the surface of the body, put pressure on him. Vector wave leads to an ordered movement elementary charges in the material, and the magnetic field acts on these charges with the Lorentz force. This force is directed in the direction of propagation. The resultant of all these forces is seen as the pressure exerted by radiation on the body. This explanation of the pressure from point of view of the wave theory. From the point of view of the quantum theory of light pressure to the surface due to the fact that each photon in a collision with the surface gives her a momentum.

Let the light falls on the surface normal. If the unit of time (t = 1 s) per unit area (S = 1m²) of the surface of the body sets the N photons, then the reflection coefficient

of light from the surface ρ - N photons reflected, and (1 - ρ) N - absorbed. Every photon absorbed by the surface, give her momentum

and each reflected

Light pressure on the surface is equal to the momentum that is transmitted to the surface in 1 s by N photons:

where - irradiance - the energy of all the photons incident on a unit area per unit time, - volumetric energy density.

Pressure light at normal incidence

Pressure of light, if the light incident at an angle i:

The number of photons per unit volume (concentration of photons)

[n] = m^-3.

The number of photons incident per unit time per unit area:

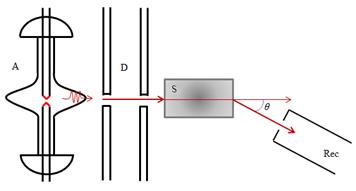
The Compton effect

Another effect, which manifests particle properties of light, is the effect of A. Compton (1923), is to change the wavelength of scattered light atoms (paraffin, graphite, boron) X-rays.

Diagram of experiments Compton: monochromatic X-rays generated by a X -ray tube passes through the aperture A and narrow beam directed to a light scattering material S. The rays scattered through an angle θ, X -rays detected receiver Rec. - X-ray spectrograph, which measures the wavelength of the scattered X -rays. Compton's experiments have shown that the wavelength λ' scattered light is larger than the wavelength λ of the incident light, the difference λ'- λ depends only on the scattering angle θ:

- Compton wavelength, determined by the mass of the substance.
Explanation of the Compton effect is given on the basis of quantum ideas about the nature of light.

In light atoms loosely bound electrons and nuclei, so the electrons can be considered free. Then Compton effect - the result of an elastic collision X-ray photons with free electrons. For an elastic collision the law of conservation of energy and momentum conservation is performed.

The law of conservation of energy for the Compton effect (the energy of the system is equal to the interaction energy of the system after the interaction)

where hν - the energy of the incident photon,

m₀c - the energy of an electron at rest,

hν’ - the energy of the scattered photon

hν + m₀c - energy to the interaction.

The law of conservation of momentum for the Compton effect:

- The momentum of the incident photon;

p'- momentum of the electron impact;

- Momentum of the scattered photon.

Mass of a relativistic particle

Energy

                                     (1)  

                                                            (2)

Square it, and recognize that

                                                    (3)

From (2)

                                                          (4)

Comparing (3) and (4) we get:

Multiply by and get

We take into account

therefore,

Wave-particle duality of light properties
In such experiments as interference, diffraction, polarization, dispersion, the wave properties of light and light are used to describe the characteristics of the wave: λ, ν. In the effects of quantum optics: thermal radiation, photoelectric effect, photochemical action of light, the light pressure, the Compton effect, the light behaves as a particle and its descriptions are used corpuscular characteristics: mass, momentum. Development of optics, the whole set of optical phenomena show that the continuity characteristic of the electromagnetic field of the light wave should not be opposed properties of the discrete characteristic of the photons. Light has a sophisticated particle-wave properties: it has both a wave and quantum properties - the wave-particle duality (duality) of the properties of light.
Communication and wave properties of light reflect formulas for the energy, momentum and mass of the photon: