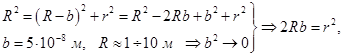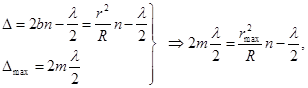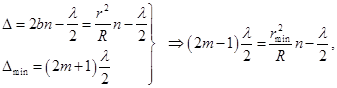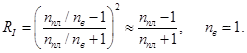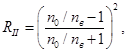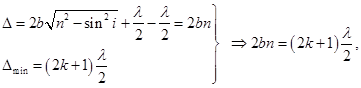Fringes of equal thickness. Newton's rings. Coated optics. The diffraction of light. The diffraction of light and the conditions for its observation. Huygens-Fresnel principle.

main

To the list of lectures

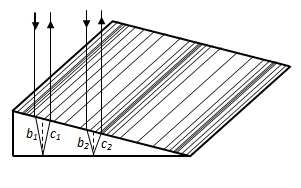
2. Fringes of constant thickness.

Assume that the plate thickness is not constant (~ b, n = const).

Then in all the places of the plate, where the thickness b, and, hence, the path difference Δ are the same, there is one and the same result of the interference. This means that along some dark or light interference fringe formed on the surface, the thickness of the plates is the same.

Fringes of constant thickness located on the surface of the plate. When observed in white light bands will be painted so that the surface contains all the colors of the rainbow. Example bands of equal thickness: oil stains, soap film, etc.

3. Newton's rings.

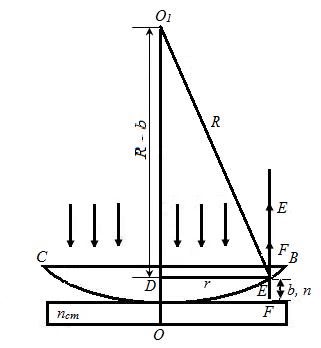
Newton's rings - an example of fringes of constant thickness,. They occur when light is reflected from the contact with each other plane-thick glass plate and a plano-convex lens with a large radius of curvature. The role of a thin film of variable thickness b, the surface from which are reflected of the coherent wave, is a gap between the plate and the lens. Let the refractive index of the gap is n, the thickness at the point E is b. Parallel beam of light falls normally (i₁ = 0 °) on a flat surface BC lenses and reflected from the top and bottom of the gap (from point E and F).

Find the radius of Newton's rings r.

The optical path difference between the rays reflected from the top and bottom of the gap is

(n < n_gl)

λ/2 accounts for the phase shift on π by reflection from an optically denser medium in point F.

From the triangle О₁DЕ follows

Then

radius of Newton's rings for the reflected light..

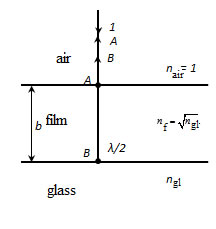
radius of Newton's rings for transmitted light.

4. Coated optics.

The possibility of reducing the reflected light from the interference in thin films are widely used in modern optical devices (cameras, binoculars, periscopes, etc.). To do this on the front surface of the available lenses and prisms are applied in a thin transparent film, the absolute refractive index n_f less n_lens. The film thickness is chosen in such a way that carried out the interference minimum reflection for light with λ = 5.5 • 10^-7 m, corresponding to the maximum sensitivity of the human eye (green). Such optics called coated. In reflected light, coated lenses appear colored in purple, as they are noticeably reflect only red and blue-violet light.
The most complete mutual extinction of the light waves reflected from the top and bottom surfaces of the coated film on the lens, is the case of equality of the intensities of these waves, ie, with approximate equality reflection coefficients. When i₁ = 0

Therefore, the optimal value n_f

The minimum thickness of the film is from the condition Δ:

b_min при k = 0

b min

The diffraction of light.
§ 1 of the diffraction of light and the conditions for its observation.
Huygens-Fresnel principle

If on the way of the light waves are opaque bodies or screens with apertures, then rough observations show that these bodies, form a region of shadows. This area may be outlined geometrically, assuming that light travels in straight lines, the light rays are straight lines.

A more detailed observation shows that the light waves come into the geometric shadow, and the border between areas of light and shadow appear alternating high and low light, indicating some redistribution of light energy at this boundary. This rounding light waves borders opaque bodies to form an interference energy redistribution on different areas called diffraction waves. (Or, a phenomenon that occurs when light in a medium with sharp irregularities, called diffraction of light.)

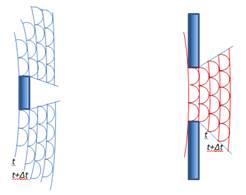
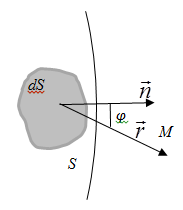
Diffraction phenomenon can be explained by using the Huygens principle: each point of the space to which comes a wave motion (ie, the wave front) is a source of secondary waves, the envelope which gives the position of the wave front in the next moment
time. In a homogeneous medium secondary waves will be of a hemisphere, the direction of propagation of the secondary waves coincides with the direction of the primary wave.
Problem of the distribution of energy along the wave front can be solved using the Huygens-Fresnel principle:

a) Huygens' principle, b) the sources of secondary waves are coherent, c) dA amplitude oscillations excited at p. M secondary sources proportional to the ratio of the area dS of the wave surface area S to the distance r from it to point M, and depends on the angle α between the external normal to the wave surface and the direction of the element dS at M.

(1)

K(φ) - proportionality factor depending on the angle φ.
When φ = 0 K(φ) = max, at φ = π/2 K(φ) = 0.

The resulting field in point M is a superposition of waves (1), taken for the entire wave surface:

(2)

-analytical account of the principle of Huygens - Fresnel.