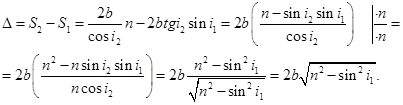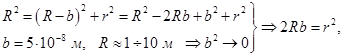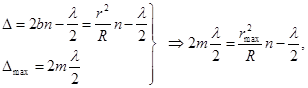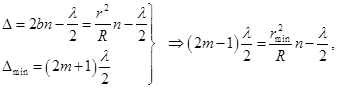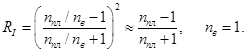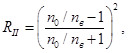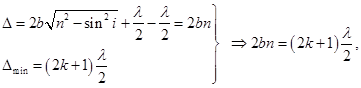Wave optics. Interference of light. Coherence and monochromatic light waves. Interference of light in thin plane-parallel plate. Fringes of equal inclination

main

To the list of lectures

OPTICS
Wave Optics
Interference of light
§ 1 The coherence and monochromatic light waves

Let the two waves of the same frequency, superimposed on each other, excited at some point in space vibrations of the same direction:

The amplitude of the resulting oscillation at a given point is given by

Initial phase

If the phase difference of the excited oscillations remains constant over time, the waves are called coherent.

The quantity

is called the optical path difference and is equal to the the difference optical length

.Optical length S of the wave is the product of a geometric path to the refractive index n:

where n - refractive index shows how many times the speed of light in a vacuum (the speed of light c = 3 ? 10⁸ m/s), more than the speed of light in a given medium – v_ph - the phase velocity.

The intensity of the wave I is proportional to the square of the amplitude I ~ A², hence

because for coherent waves, depending on the difference optical path Δ at some points will light amplification, and in others - it weakening.

If I₁ = I₂, then

I_max =4I₁,

I_min =0,

ie will be a redistribution of intensity (energy) waves in space.

Redistribution of light flux in space, in which at some points there are maximums and minimums in other intensity is called interference.

A necessary condition for the interference of waves is their coherence. However, due to the transverse electromagnetic waves their coherence condition is not sufficient to produce an interference pattern. Necessary, in coherence to vibrations vectors

of electromagnetic fields, the interfering waves occurred along the same or a similar direction, ie requires that the interfering waves propagating in the same direction and the plane

of the waves were close.

Coherent are monochromatic waves - unlimited in space of one specific wavelength and strictly constant frequency. Because no real power does not strictly monochromatic light, the waves emitted by any source of light other than a laser, are incoherent. Therefore, the experiment did not observe the interference of light from independent sources of light, such as the two bulbs.

§2 The interference of light in thin plane-plates

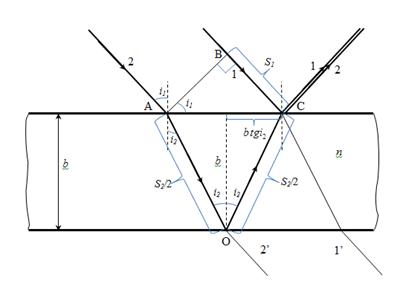
Consider a plane-parallel glass (or transparent) plate n = 1.5, thickness b (temporal coherence condition will be satisfied if that is for λ₀ = 5·10^-7 м and Δλ= 20 Å и b =6·10^-8 м). The plate is at an angle i plane monochromatic wave. The plate is in the air n_air = 1.

The incident wave is partially reflected (∼5°) from the upper surface of the plate (beam 1) and partially refracted (beam AO). Refracted wave, reaching the bottom of the plate, and partially reflected (ray ОС), and partially refracted (beam 2'). The same thing happens on the upper surface of the plate at the point with the ray ОС, and the refracted wave (beam 2) is superimposed to the wave directly reflected from the top surface (beam 1). These two waves are coherent. The result of the interference depends on the Δ - optical path difference.

The path difference acquired by the beams 1 and 2 before they meet in that C is

In geometrical optics, we know the law of refraction

of trigonometry

Then

In calculating the phase difference Δφ between oscillations in the beams 1 and 2 need besides the optical path difference Δ into account the phase change on reflection in p. A Since in p. A that there is a reflection from the interface between the optically less dense medium to an optically denser medium (n₂ >n₁, because n_glass> 1), the phase of the wave changes in A on π. In that reflection occurs on the boundary between the media, the optically denser medium to an optically less dense, so that the phase change in p. O not occurs.

Thus, the phase change in p. A can be accounted for by adding to Δ (or subtracted from) the half wavelength in a vacuum - λ / 2. Then, finally

- The optical path difference for the interference of the reflected beams 1 and 2.

- The optical path difference for the interference of passing rays 1'and 2'

§ 3 Constant inclination fringes.
Fringes of constant thickness
Newton's rings.
Coated optics

Constant inclination fringes.

Assuming for a plane-parallel plate (b = const, n = const) fall under and the two light waves. Then each point taken on the surface of the plate, will come two reflected waves, one - from a wave a, another – from a wave b. The difference of the interfering beams in point C to wave a is equal Δ₁, and for a wave b is Δ₂. Let , and .Because b= const, ,then from each point С₁, С₂, С₃, ... of the plates come the same radiation, so no interference fringes on the surface of the plate is not (the wafer surface will have the same luminosity as a function of the values ??of Δ₁ - Δ₂). On a screen located in the focal plane of the lens can be observed bright bands corresponding to wave a, and the dark, the corresponding wave b. If angles of incidence take all possible values??, the screen are obtained Interference s bands, each of which corresponds to a value of the angle of incidence i₁ - constant inclination fringes.

They say that the constant inclination fringes is localized at infinity, because for their observation needs screen located in the focal plane of the lens. (In the focal plane parallel rays incident on the lens, going to a point.)

Example inclination fringes - hologram on travel tickets, labels, etc.

2. Fringes of constant thickness.

Assume that the plate thickness is not constant (~ b, n = const).

Then in all the places of the plate, where the thickness b, and, hence, the path difference Δ are the same, there is one and the same result of the interference. This means that along some dark or light interference fringe formed on the surface, the thickness of the plates is the same.

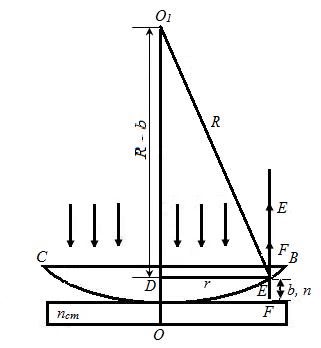
Fringes of constant thickness located on the surface of the plate. When observed in white light bands will be painted so that the surface contains all the colors of the rainbow. Example bands of equal thickness: oil stains, soap film, etc.

3. Newton's rings.

Newton's rings - an example of fringes of constant thickness,. They occur when light is reflected from the contact with each other plane-thick glass plate and a plano-convex lens with a large radius of curvature. The role of a thin film of variable thickness b, the surface from which are reflected of the coherent wave, is a gap between the plate and the lens. Let the refractive index of the gap is n, the thickness at the point E is b. Parallel beam of light falls normally (i₁ = 0 °) on a flat surface BC lenses and reflected from the top and bottom of the gap (from point E and F).

Find the radius of Newton's rings r.

The optical path difference between the rays reflected from the top and bottom of the gap is

(n < n_gl)

λ/2 accounts for the phase shift on π by reflection from an optically denser medium in point F.

From the triangle О₁DЕ follows

Then

radius of Newton's rings for the reflected light.

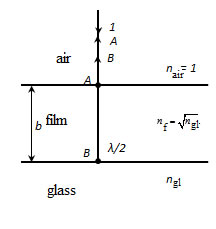
radius of Newton's rings for transmitted light.

4. Coated optics.

The possibility of reducing the reflected light from the interference in thin films are widely used in modern optical devices (cameras, binoculars, periscopes, etc.). To do this on the front surface of the available lenses and prisms are applied in a thin transparent film, the absolute refractive index n_f less n_lens. The film thickness is chosen in such a way that carried out the interference minimum reflection for light with λ = 5.5 • 10^-7 m, corresponding to the maximum sensitivity of the human eye (green). Such optics called coated. In reflected light, coated lenses appear colored in purple, as they are noticeably reflect only red and blue-violet light.
The most complete mutual extinction of the light waves reflected from the top and bottom surfaces of the coated film on the lens, is the case of equality of the intensities of these waves, ie, with approximate equality reflection coefficients. When i₁ = 0

Therefore, the optimal value n_f

The minimum thickness of the film is from the condition Δ:

b_min at k = 0

b min