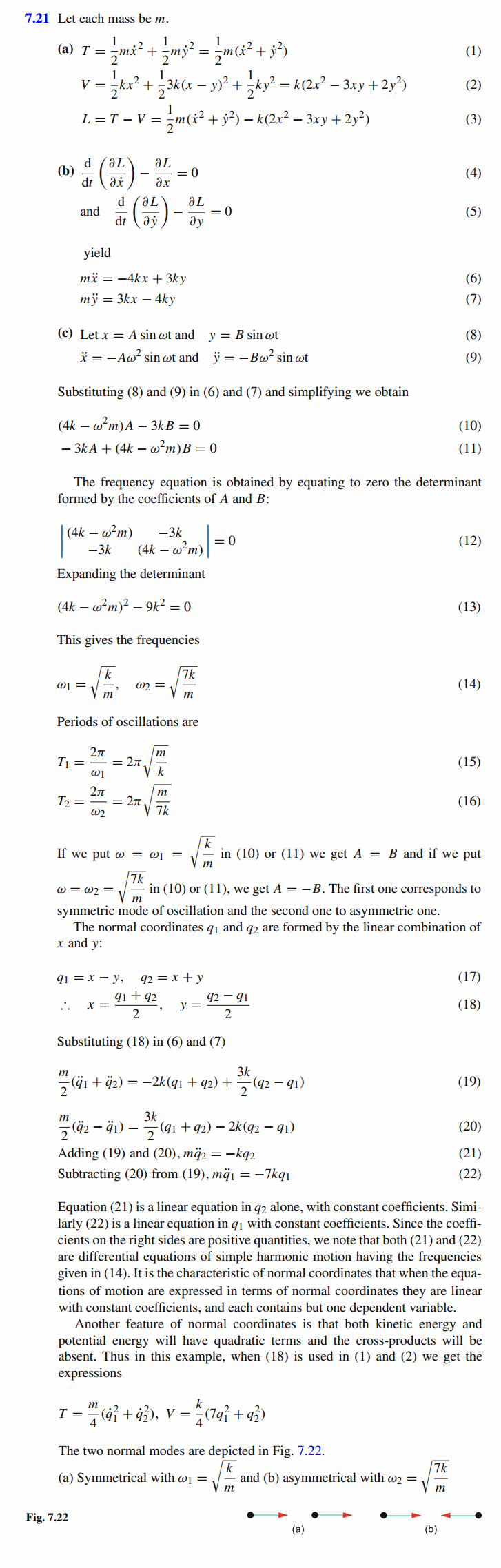
Consider the system consisting of two identical masses that can move horizontally, joined with springs as shown in Fig. 7.4. Let x,y be the horizontal displacements of the two masses from their equilibrium positions. (a) Find the kinetic and potential energies of the system and deduce the Lagrangian. (b) Show that Lagrange's equation gives the coupled linear differential equations mx··=-4kx+3ky and my··=3kx-4ky (c) Find the normal modes of oscillation of this system and their period of oscillation.
Solution:
|

