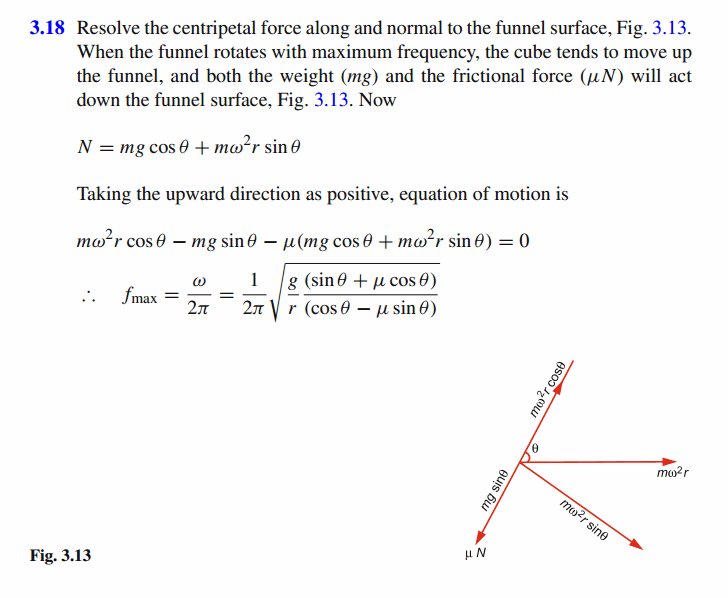
A small cube placed on the inside of a funnel rotates about a vertical axis at a constant rate of f rev/s. The wall of the funnel makes an angle T with the horizontal (Fig. 3.5). If the coefficient of static friction is n and the centre of the cube is at a distance r from the axis of rotation, show that the largest frequency for which the block will not move with respect to the funnel is fmax = 1/2pi·sqrt(g(sinT+ncosT)/r(cosT-nsinT)) |
| New search. (Also 5349 free access solutions) |