A pendulum of length l and mass m is mounted on a block of mass M . The block can move freely without friction on a horizontal surface as shown in Fig. 7.7. (a) Show that the Lagrangian for the system is L=((M+m)/2)(x·)^2+mlcosT(x·)(T·)+m/2l^2(T·)^2+mglcosT (b) Show that the approximate form for this Lagrangian, which is applicable for a small amplitude swinging of the pendulum, is L=((M+m)/2)(x·)^2+ml(x·)T·+m/2(l^2)(T·)^2+mgl(1-T^2/2) Find the equations of motion that follow from the simplified Lagrangian obtained in part (b), (d) Find the frequency of a small amplitude oscillation of the system. [University of Manchester 2006]
Solution:
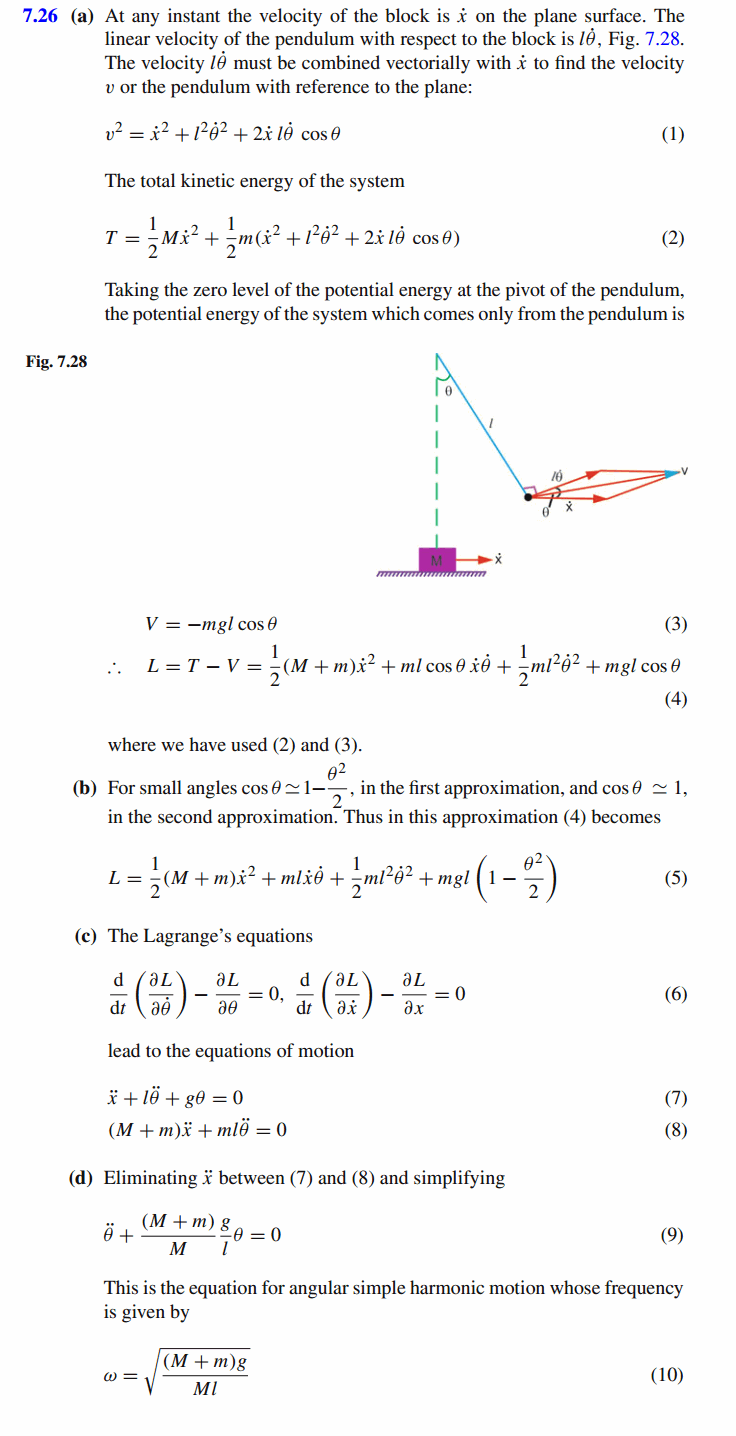
|

