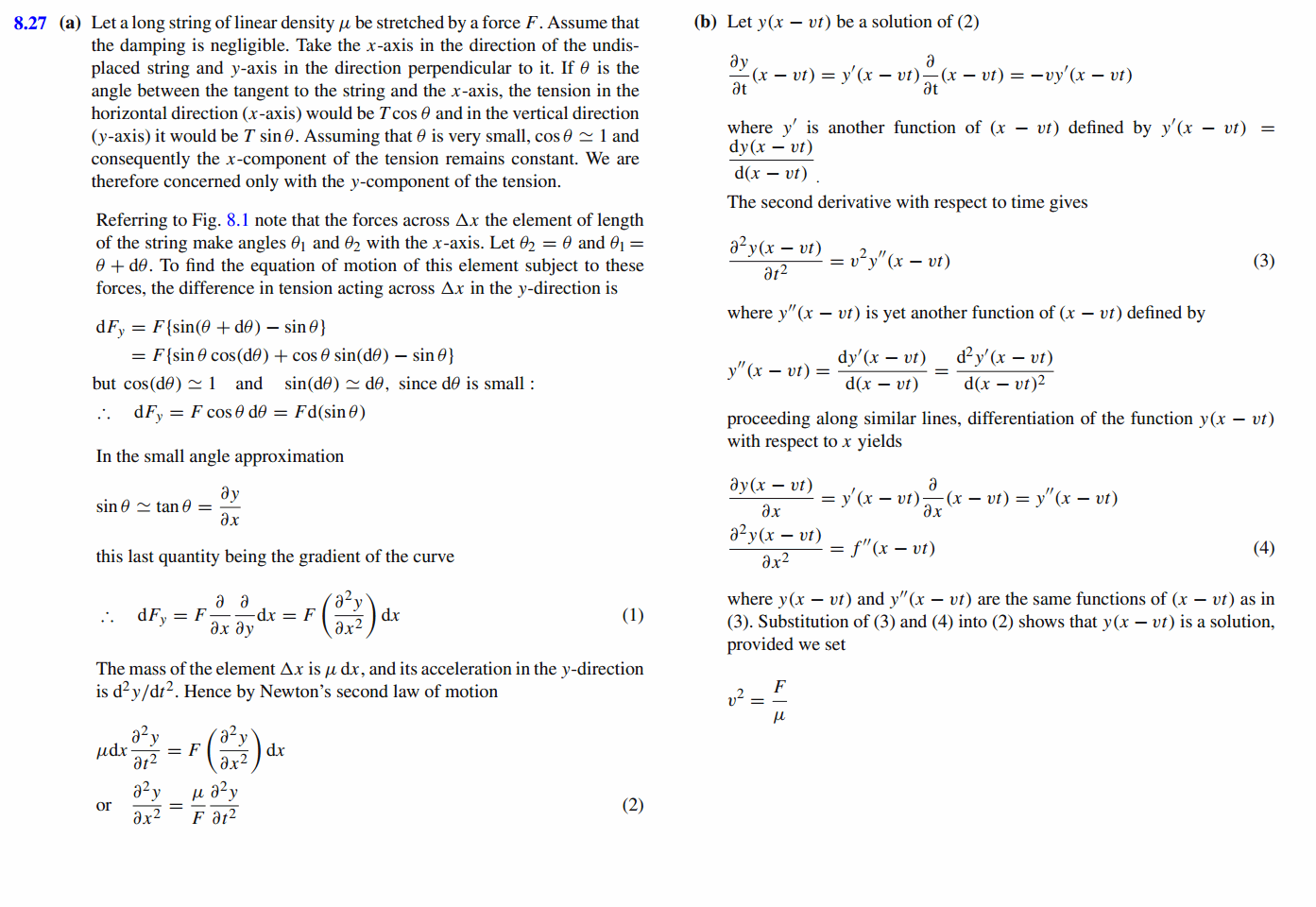
(a) Consider a small segment of a string upon which a wave pulse is travelling. Using this diagram, or otherwise, show that the wave equation for transverse waves on a stretched string is d^2y/dx^2=(n/F)d^2y/dt^2 where n is the mass per unit length and F is the tension. (b) Show that the wave function representing a wave travelling in the positive x-direction, y(x-vt), is a solution of the wave equation. Obtain an expression for the velocity, v, of the wave (Fig. 8.1). |
| New search. (Also 5349 free access solutions) |